Post History

Please note that the text refers to the *integral*, or the mathematical evaluation through integration which, indeed, cannot be obtained. But that doesn't mean you can't obtain the Laplace transfer function directly. A hypothetical RLC filter with a negative resistor is very much possible. In fact, it can be made in practice with emulated elements, and its transfer function will give a pole in the RHP:

$$H(s)=\dfrac{\dfrac{1}{LC}}{s^2-\dfrac{1}{RC}s+\dfrac{1}{LC}}$$

This can be obtaind by considering the raw Laplace equivalents of the elements. Is it unstable? Yes. Can it be done practically? Yes, here is the concept of it:

![-RLC](https://electrical.codidact.com/uploads/pQW4mrVmZbuBzZvkvG8kPjtA)

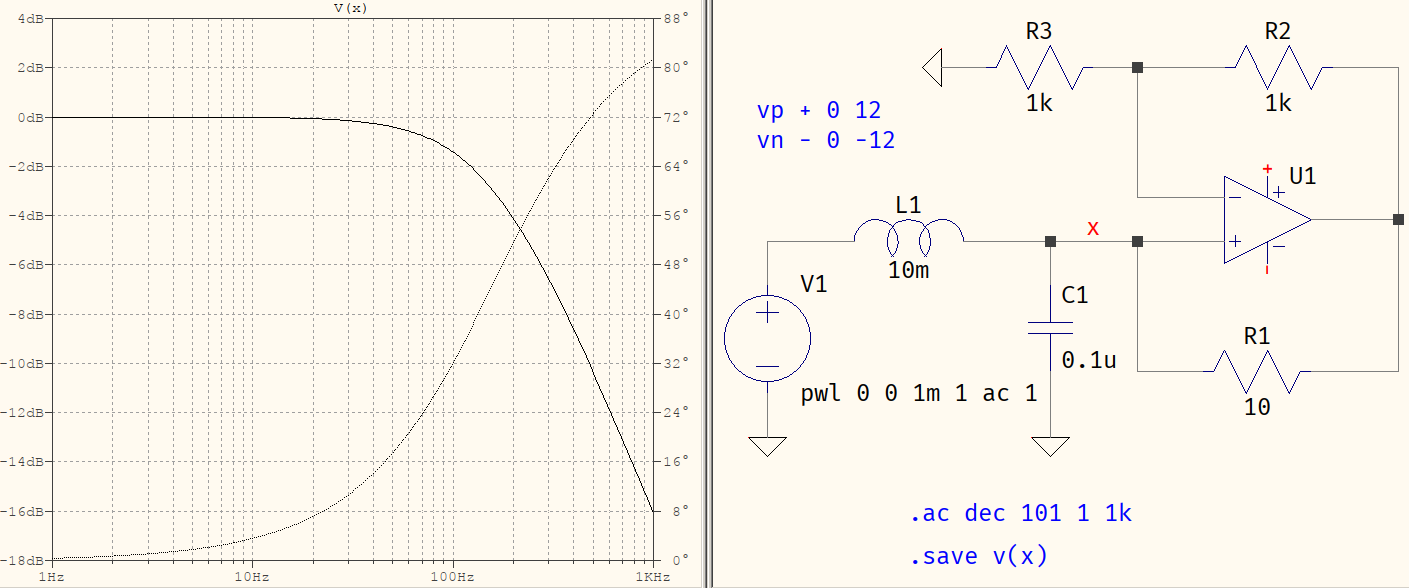
You can clearly see the phase going up. Does that violate the textbook? No -- the textbook only picks on the mathematical aspect (Olin says it better: semantics).