Post History
#3: Post edited
- It is well known that there is a linear relationship between the BJT collector and base current (Ic = beta x Ib). It would be interesting to explain it in an intuitive way. The power of such a "philosophical" approach is that it can explain various specific implementations.
- The idea
- --------
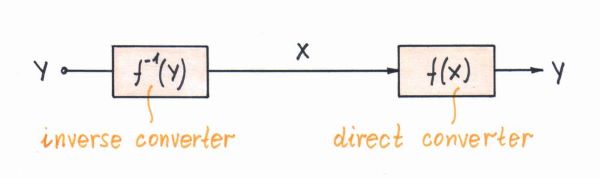
- In the BJT, the base-emitter voltage controls the collector current in an exponential manner. So, if we directly drive the base-emitter junction by a perfect voltage source, the bare transistor can be thought as an "antilog voltage-to-current converter".
If we drive the base-emitter junction through a resistor or by a perfect current source, we will control indirectly the base-emitter voltage... and it will control the collector current as usual. So the base-emitter junction serves here as a "log current-to-voltage converter".- The conclusion is that, in this arrangement, there are two cascaded (reverse and direct) non-linear convertors. .. and the overall relation between the output quantity (collector current) and input quantity (base current) of this "aggregate" is linear - Ic = beta x Ib.
- 
- Conceiving the idea
- -------------------
- I came to this insight after analyzing in detail the BJT current mirror and so I realized the role of the input transistor T1 (the so-called "active diode"). Here is the evolution of my reasoning...
- 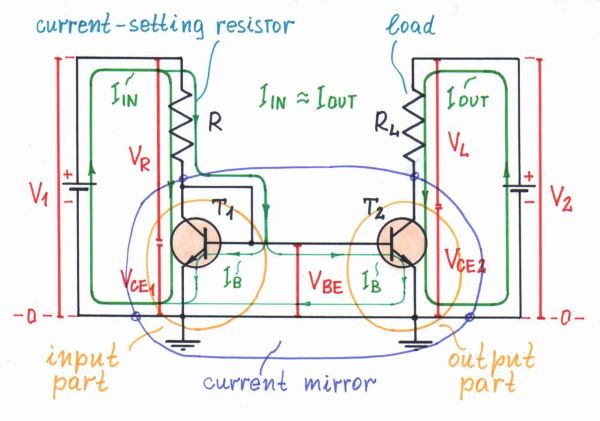
The key point here is that it is not the "active diode" that makes the current mirror... and it is not the one that provides the linearity; *both they are provided by the humble second transistor*. Indeed, it is not exactly a *current mirror*; it is rather a linear *current amplifier* since Ic = beta x Ib = beta x Iin. That is why the input "active diode" is connected in parallel to the base-emitter "diode" - to divert the excess input current and leave only the small base current so Ic = Iin. It is not connected to ensure the input log conversion since the second transistor does it itself by its base-emitter junction; it is connected to equalize the input and output currents.- Generalization
- --------------
- This conclusion (linear relation) applies to *every transconductance converter which input behaves as the opposite converter*... or, in other words, it applies to every 2-port voltage-to-current (transconductance) converter which input behaves as a 1-port current-to-voltage converter. In our example, the BJT is an antilog transconverter (a "transdiode" controlled by voltage at one place that produces current at other place) which input is a log converter (a diode controlled by current that produces voltage at the same place).
- Note the ordinary transconductance (voltage-to-current) converter does not obey this idea since it can have a true voltage input (with extremely high resistance). Its input must be conductive... and with the opposite relation. So we can emulate it by connecting (embedding) an external element (diode) in parallel to the input. For example, imagine the MOSFET transfer characteristic was exponential like BJT. The problem is that its input is extremely high resistive (open circuit). So we can connect a diode in parallel to the gate-source input to immitate the BJT base-emitter junction; thus the whole combination will act as a "BJT" with a linear relation Id = k.Iin.
- Variations
- ----------
- It seems the opposite is possible as well but not so useful - to make a voltage-to-voltage converter by cascading a voltage-to-current and current-to-voltage converters. Interesting... it seems the op-amp inverting amplifier is such an example where R1 acts as the input voltage-to-current converter and the rest (R2 and the op-amp) acts as a current-to-voltage converter (transimpedance amplifier). The transfer ratio is linear. But it would be linear even we replace the ohmic R1 and R2 with nonlinear resistors (e.g., diodes)... a quite odd and somehow conflicting configuration... In this case we will apply input voltage and will obtain output voltage... and the relation between them will be linear.
- -----
- I would like to know your opinion if this is a reliable viewpoint at the bipolar transistor and its current transfer characteristic I = f(Ib).
- It is well known that there is a linear relationship between the BJT collector and base current (Ic = beta x Ib). It would be interesting to explain it in an intuitive way. The power of such a "philosophical" approach is that it can explain various specific implementations.
- The idea
- --------
- In the BJT, the base-emitter voltage controls the collector current in an exponential manner. So, if we directly drive the base-emitter junction by a perfect voltage source, the bare transistor can be thought as an "antilog voltage-to-current converter".
- If we drive the base-emitter junction by a voltage source through a resistor or by a perfect current source, we will control indirectly the base-emitter voltage... and it will control the collector current as usual. So the base-emitter junction serves here as a "log current-to-voltage converter".
- The conclusion is that, in this arrangement, there are two cascaded (reverse and direct) non-linear convertors. .. and the overall relation between the output quantity (collector current) and input quantity (base current) of this "aggregate" is linear - Ic = beta x Ib.
- 
- Conceiving the idea
- -------------------
- I came to this insight after analyzing in detail the BJT current mirror and so I realized the role of the input transistor T1 (the so-called "active diode"). Here is the evolution of my reasoning...
- 
- The key point here is that it is not the "active diode" that makes the current mirror... and it is not the one that provides the linearity; *both they are provided by the humble second transistor*. Indeed, it is not exactly a *current follower*; it is rather a linear *current amplifier* since Ic = beta x Ib = beta x Iin. That is why the input "active diode" is connected in parallel to the base-emitter "diode" - to divert the excess input current and leave only the small base current so Ic = Iin. It is not connected to ensure the input log conversion since the second transistor does it itself by its base-emitter junction; it is connected to equalize the input and output currents.
- Generalization
- --------------
- This conclusion (linear relation) applies to *every transconductance converter which input behaves as the opposite converter*... or, in other words, it applies to every 2-port voltage-to-current (transconductance) converter which input behaves as a 1-port current-to-voltage converter. In our example, the BJT is an antilog transconverter (a "transdiode" controlled by voltage at one place that produces current at other place) which input is a log converter (a diode controlled by current that produces voltage at the same place).
- Note the ordinary transconductance (voltage-to-current) converter does not obey this idea since it can have a true voltage input (with extremely high resistance). Its input must be conductive... and with the opposite relation. So we can emulate it by connecting (embedding) an external element (diode) in parallel to the input. For example, imagine the MOSFET transfer characteristic was exponential like BJT. The problem is that its input is extremely high resistive (open circuit). So we can connect a diode in parallel to the gate-source input to immitate the BJT base-emitter junction; thus the whole combination will act as a "BJT" with a linear relation Id = k.Iin.
- Variations
- ----------
- It seems the opposite is possible as well but not so useful - to make a voltage-to-voltage converter by cascading a voltage-to-current and current-to-voltage converters. Interesting... it seems the op-amp inverting amplifier is such an example where R1 acts as the input voltage-to-current converter and the rest (R2 and the op-amp) acts as a current-to-voltage converter (transimpedance amplifier). The transfer ratio is linear. But it would be linear even we replace the ohmic R1 and R2 with nonlinear resistors (e.g., diodes)... a quite odd and somehow conflicting configuration... In this case we will apply input voltage and will obtain output voltage... and the relation between them will be linear.
- -----
- I would like to know your opinion if this is a reliable viewpoint at the bipolar transistor and its current transfer characteristic I = f(Ib).
#2: Post edited
- It is well known that there is a linear relationship between the BJT collector and base current (Ic = beta x Ib). It would be interesting to explain it in an intuitive way. The power of such a "philosophical" approach is that it can explain various specific implementations.
- The idea
- --------
In the BJT, the base-emitter voltage controls the collector current in an exponential manner. So if we directly drive the base-emitter junction by a perfect voltage source, the bare transistor can be thought as an "antilog voltage-to-current converter".- If we drive the base-emitter junction through a resistor or by a perfect current source, we will control indirectly the base-emitter voltage... and it will control the collector current as usual. So the base-emitter junction serves here as a "log current-to-voltage converter".
- The conclusion is that, in this arrangement, there are two cascaded (reverse and direct) non-linear convertors. .. and the overall relation between the output quantity (collector current) and input quantity (base current) of this "aggregate" is linear - Ic = beta x Ib.
- 
- Conceiving the idea
- -------------------
- I came to this insight after analyzing in detail the BJT current mirror and so I realized the role of the input transistor T1 (the so-called "active diode"). Here is the evolution of my reasoning...
- 
- The key point here is that it is not the "active diode" that makes the current mirror... and it is not the one that provides the linearity; *both they are provided by the humble second transistor*. Indeed, it is not exactly a *current mirror*; it is rather a linear *current amplifier* since Ic = beta x Ib = beta x Iin. That is why the input "active diode" is connected in parallel to the base-emitter "diode" - to divert the excess input current and leave only the small base current so Ic = Iin. It is not connected to ensure the input log conversion since the second transistor does it itself by its base-emitter junction; it is connected to equalize the input and output currents.
- Generalization
- --------------
- This conclusion (linear relation) applies to *every transconductance converter which input behaves as the opposite converter*... or, in other words, it applies to every 2-port voltage-to-current (transconductance) converter which input behaves as a 1-port current-to-voltage converter. In our example, the BJT is an antilog transconverter (a "transdiode" controlled by voltage at one place that produces current at other place) which input is a log converter (a diode controlled by current that produces voltage at the same place).
Note the ordinary transconductance (voltage-to-current) converter does not obey this idea since it can have a true voltage input (with extremely high resistance). Its input must be conductive... and with the opposite relation. So we can emulate it by connecting an external element (diode) in parallel to the input. For example, imagine the MOSFET transfer characteristic was exponential like BJT. The problem is that its input is extremely high resistive (open circuit). So we can connect a diode in parallel to the gate-source input to immitate the BJT base-emitter junction; thus the whole combination will act as a BJT with linear relation Id = k.Iin.- Variations
- ----------
- It seems the opposite is possible as well but not so useful - to make a voltage-to-voltage converter by cascading a voltage-to-current and current-to-voltage converters. Interesting... it seems the op-amp inverting amplifier is such an example where R1 acts as the input voltage-to-current converter and the rest (R2 and the op-amp) acts as a current-to-voltage converter (transimpedance amplifier). The transfer ratio is linear. But it would be linear even we replace the ohmic R1 and R2 with nonlinear resistors (e.g., diodes)... a quite odd and somehow conflicting configuration... In this case we will apply input voltage and will obtain output voltage... and the relation between them will be linear.
- -----
- I would like to know your opinion if this is a reliable viewpoint at the bipolar transistor and its current transfer characteristic I = f(Ib).
- It is well known that there is a linear relationship between the BJT collector and base current (Ic = beta x Ib). It would be interesting to explain it in an intuitive way. The power of such a "philosophical" approach is that it can explain various specific implementations.
- The idea
- --------
- In the BJT, the base-emitter voltage controls the collector current in an exponential manner. So, if we directly drive the base-emitter junction by a perfect voltage source, the bare transistor can be thought as an "antilog voltage-to-current converter".
- If we drive the base-emitter junction through a resistor or by a perfect current source, we will control indirectly the base-emitter voltage... and it will control the collector current as usual. So the base-emitter junction serves here as a "log current-to-voltage converter".
- The conclusion is that, in this arrangement, there are two cascaded (reverse and direct) non-linear convertors. .. and the overall relation between the output quantity (collector current) and input quantity (base current) of this "aggregate" is linear - Ic = beta x Ib.
- 
- Conceiving the idea
- -------------------
- I came to this insight after analyzing in detail the BJT current mirror and so I realized the role of the input transistor T1 (the so-called "active diode"). Here is the evolution of my reasoning...
- 
- The key point here is that it is not the "active diode" that makes the current mirror... and it is not the one that provides the linearity; *both they are provided by the humble second transistor*. Indeed, it is not exactly a *current mirror*; it is rather a linear *current amplifier* since Ic = beta x Ib = beta x Iin. That is why the input "active diode" is connected in parallel to the base-emitter "diode" - to divert the excess input current and leave only the small base current so Ic = Iin. It is not connected to ensure the input log conversion since the second transistor does it itself by its base-emitter junction; it is connected to equalize the input and output currents.
- Generalization
- --------------
- This conclusion (linear relation) applies to *every transconductance converter which input behaves as the opposite converter*... or, in other words, it applies to every 2-port voltage-to-current (transconductance) converter which input behaves as a 1-port current-to-voltage converter. In our example, the BJT is an antilog transconverter (a "transdiode" controlled by voltage at one place that produces current at other place) which input is a log converter (a diode controlled by current that produces voltage at the same place).
- Note the ordinary transconductance (voltage-to-current) converter does not obey this idea since it can have a true voltage input (with extremely high resistance). Its input must be conductive... and with the opposite relation. So we can emulate it by connecting (embedding) an external element (diode) in parallel to the input. For example, imagine the MOSFET transfer characteristic was exponential like BJT. The problem is that its input is extremely high resistive (open circuit). So we can connect a diode in parallel to the gate-source input to immitate the BJT base-emitter junction; thus the whole combination will act as a "BJT" with a linear relation Id = k.Iin.
- Variations
- ----------
- It seems the opposite is possible as well but not so useful - to make a voltage-to-voltage converter by cascading a voltage-to-current and current-to-voltage converters. Interesting... it seems the op-amp inverting amplifier is such an example where R1 acts as the input voltage-to-current converter and the rest (R2 and the op-amp) acts as a current-to-voltage converter (transimpedance amplifier). The transfer ratio is linear. But it would be linear even we replace the ohmic R1 and R2 with nonlinear resistors (e.g., diodes)... a quite odd and somehow conflicting configuration... In this case we will apply input voltage and will obtain output voltage... and the relation between them will be linear.
- -----
- I would like to know your opinion if this is a reliable viewpoint at the bipolar transistor and its current transfer characteristic I = f(Ib).
#1: Initial revision
Why does the collector current depend linearly on the base current?
It is well known that there is a linear relationship between the BJT collector and base current (Ic = beta x Ib). It would be interesting to explain it in an intuitive way. The power of such a "philosophical" approach is that it can explain various specific implementations. The idea -------- In the BJT, the base-emitter voltage controls the collector current in an exponential manner. So if we directly drive the base-emitter junction by a perfect voltage source, the bare transistor can be thought as an "antilog voltage-to-current converter". If we drive the base-emitter junction through a resistor or by a perfect current source, we will control indirectly the base-emitter voltage... and it will control the collector current as usual. So the base-emitter junction serves here as a "log current-to-voltage converter". The conclusion is that, in this arrangement, there are two cascaded (reverse and direct) non-linear convertors. .. and the overall relation between the output quantity (collector current) and input quantity (base current) of this "aggregate" is linear - Ic = beta x Ib.  Conceiving the idea ------------------- I came to this insight after analyzing in detail the BJT current mirror and so I realized the role of the input transistor T1 (the so-called "active diode"). Here is the evolution of my reasoning...  The key point here is that it is not the "active diode" that makes the current mirror... and it is not the one that provides the linearity; *both they are provided by the humble second transistor*. Indeed, it is not exactly a *current mirror*; it is rather a linear *current amplifier* since Ic = beta x Ib = beta x Iin. That is why the input "active diode" is connected in parallel to the base-emitter "diode" - to divert the excess input current and leave only the small base current so Ic = Iin. It is not connected to ensure the input log conversion since the second transistor does it itself by its base-emitter junction; it is connected to equalize the input and output currents. Generalization -------------- This conclusion (linear relation) applies to *every transconductance converter which input behaves as the opposite converter*... or, in other words, it applies to every 2-port voltage-to-current (transconductance) converter which input behaves as a 1-port current-to-voltage converter. In our example, the BJT is an antilog transconverter (a "transdiode" controlled by voltage at one place that produces current at other place) which input is a log converter (a diode controlled by current that produces voltage at the same place). Note the ordinary transconductance (voltage-to-current) converter does not obey this idea since it can have a true voltage input (with extremely high resistance). Its input must be conductive... and with the opposite relation. So we can emulate it by connecting an external element (diode) in parallel to the input. For example, imagine the MOSFET transfer characteristic was exponential like BJT. The problem is that its input is extremely high resistive (open circuit). So we can connect a diode in parallel to the gate-source input to immitate the BJT base-emitter junction; thus the whole combination will act as a BJT with linear relation Id = k.Iin. Variations ---------- It seems the opposite is possible as well but not so useful - to make a voltage-to-voltage converter by cascading a voltage-to-current and current-to-voltage converters. Interesting... it seems the op-amp inverting amplifier is such an example where R1 acts as the input voltage-to-current converter and the rest (R2 and the op-amp) acts as a current-to-voltage converter (transimpedance amplifier). The transfer ratio is linear. But it would be linear even we replace the ohmic R1 and R2 with nonlinear resistors (e.g., diodes)... a quite odd and somehow conflicting configuration... In this case we will apply input voltage and will obtain output voltage... and the relation between them will be linear. ----- I would like to know your opinion if this is a reliable viewpoint at the bipolar transistor and its current transfer characteristic I = f(Ib).


















