Post History

 Results of analysis of Hartley oscillator dont make sense

I want to find the conditions of oscillation of the following Hartley oscillator.I have attached a load (ZL) to my Hartley oscillator

![Image alt text](https://electrical.codidact.com/uploads/Sg52ZRJ87zL6LTmyvGJnh2Zg)

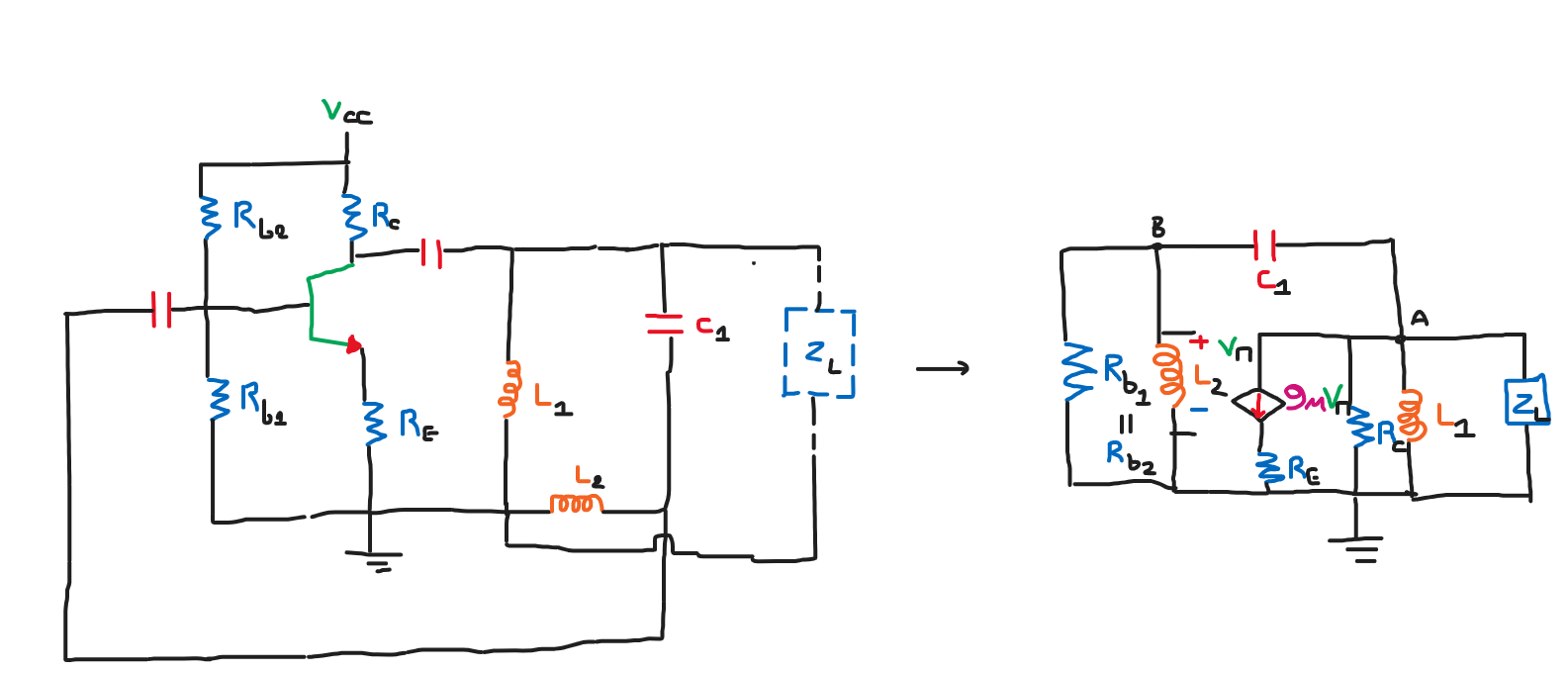
I have written KCL for nodes A,B:

For node A:

$$\frac{V_{A}}{sL_{1}} + \frac{V_{A}}{Z_{L}}+\frac{V_{A}}{R_{C}}-sC_{1}(V_{A}-V_{B})+g_{m}V_{x} = 0 \rightarrow V_{A}(Z_{L}R_{C}+sL_{1}R_{C}+sL_{1}Z_{L})-sC_{1}sL_{1}R_{C}Z_{L}(V_{B}-V_{A})+g_{m}V_{B} = 0 \rightarrow (g_{m}-sL_{1}sC_{1}R_{C}Z_{L})V_{B} = (-sL_{1}sC_{1}R_{C}Z_{L}-R_{C}Z_{L}-sL_{1}R_{C}-sL_{1}Z_{L})V_{A}$$

But $$\frac{V_{o}}{V_{in}} = 1 \rightarrow $$

$$g_{m}-sL_{1}sC_{1}R_{C}Z_{L} = -sL_{1}sC_{1}R_{C}Z_{L}-R_{C}Z_{L}-sL_{1}R_{C}-sL_{1}Z_{L} \rightarrow g_{m} = -R_{C}Z_{L} , -sL_{1}R_{C} = sL_{1}Z_{L} $$


which doesnt make any sense.Where am I doing wrong in my analysis?